The new earliest “Long Count” or “Initial Series” date on Tikal Stela 29 is transcribed as “8.12.14.8.15 13 Men 3 Zip.” We here compare it with a random date in our own Christian Civil Calendar system, hoping to bring out similarities as well as differences for the edification of the mildly curious reader. The left part of the tabulation deals with our date A.D. 1960, Thursday, June 23, and the right part with the Maya date of Stela 29. Each element of the Christian date corresponds in some way with that of the Maya date shown on the same line.
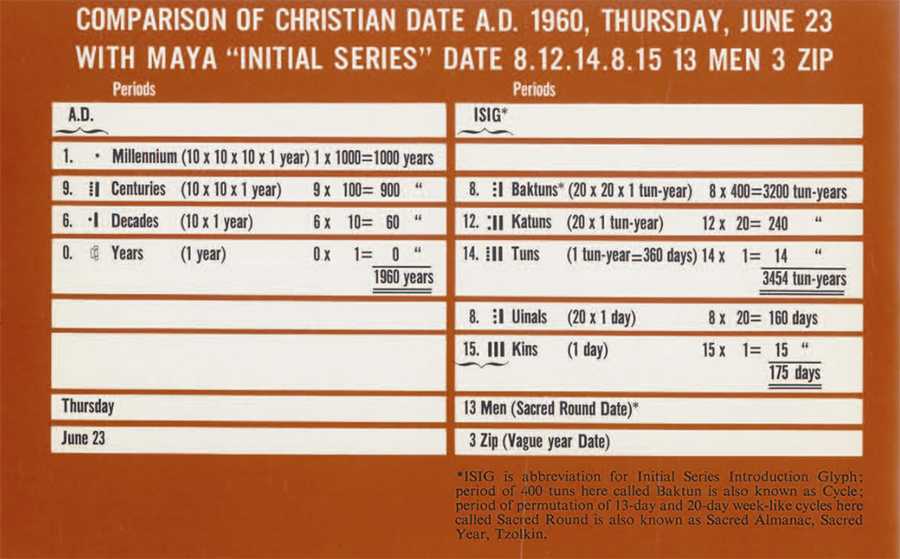
The Maya arithmetic system is based on 20, rather than the 10 of our decimal system. When we transcribe their digits into the decimal system we must often use numbers above 10 for a single digit. Hence the points marking off the five digits or terms in “8.12.14.8.15.” To make comparison more easy we use them for the Christian date also, “1.9.6.0,” so that it is clearer that the Maya Long Count “date” is a numerically expressed one, like our 1960. In the tabulations, both dates are written vertically, as was done on the stela. Then each is repeated, to the right, in the usual Maya bar-dot notation for individual terms or digits, using one bar for each five units in a digit, and one dot for each remaining single unit. In this transcription of the Christian 1.9.6.0 into the Maya bar-dot notation of a Maya sign for the zero is necessary, as it might be also in some other Maya number than that on Stela 29. The zero sign would be used wherever needed, just as with us.
We may not often think of it, but the expression A.D. 1960 involves the concept of periods of time, for which we have names, so we give them in the table (year, decade, century, millennium). The basic period for this “Christian Long Count” is the year. In the Maya Long Count it is a sort of “computing year” called tun, always consisting of 360 days (18 X 20), hence shorter than the 365 or 366-day Christian year. Just as the Christian periods increase in value by 10’s, starting with the year as the unit, the Maya periods increase by 20’s, if we start with the tun as the basic period. As a result, the highest three terms of a Maya 5-place number may cover much more ground than all four terms of the Christian counterpart. Thus the number of Stela 29 covers 3454 “tun-years,” and though the tun-year is about 5 1/4 days shorter than our average year, the period covered is much more than 1960 of our years.
The names for the higher Maya periods based on the tun are katun and baktun, corresponding to our “decade” and “century,” except that they cover more time. The baktun is sometimes called “cycle.” So the Stela 29 date is a Baktun 8 or Cycle 8 date. Most Maya dates are later, in Baktun 9.
The Maya were not content to place one at some unknown point within the current computing year or tun. So the lowest two terms give this position in terms of the single day as the basic unit, and 20’s of days as the next. These periods were called kin (day) and uinal (probably meaning “twenty”). This is the reason we have a five-term number instead of a three-term one. In a day-by-day count, on reaching 18 uinals these convert to 1 tun. The full distance covered by 8.12.14.8.15 tun-years and 175 days.
Though it was not necessary, on the stone monuments the Maya gave glyphs for the five periods after the necessary bar-dot numerals, and the latter are referred to as the “coefficients” of the respective periods. For an analogy, in the expression “1 millennium,” the millennium coefficient is 1. As the illustrations show, on Stela 29 the period glyphs for baktun, katun, and tun are bird heads; that for the uinal is a frog head; the period glyph for kin is missing, but might have been the Sun God’s head. Period glyphs, identified by position, may vary much.
At the top of the stela, the text begins with the “Initial Series Introducing Glyph,” which corresponds with the “A.D.” before 1960 in that it tells us that the count is forward from a standard fixed point in past time, used for nearly all other “Initial Series” dates. This base date, 4 Ahau 8 Cumku, was calculated back into the mythical past, differing in this respect from our base-date or era, which is the historically experienced birth of Christ.
This expression “4 Ahau” places one at the 4th day of a week-like cycle of 13 numbered days, and at the 20th day (called Ahau) of another week-like cycle of twenty named days. Either of these “week dates” corresponds fairly well with our 7-day week, and positions in both were regularly recorded. Running concurrently they form a “Sacred Round” period of 260 days, after which positions in both will again be the same. The expression “8 Cumku” means the 8th day of the month called Cumku, in a “vague year” of 365 days. In this second sort of Maya year there were eighteen 20-day named months and an extra 5-day period. Combined with the two “weeks” this set up a 52-year “Calendar Round” permutation, which the Maya shared with other Mesoamericans.
Knowing the Calendar Round date at the Initial Series base, which is implied by the Introducing Glyph, one may calculate what Calendar Round date must be reached by a Long Count number such as the 8.12.14.8.15 of Stelka 29. Though it is almost entirely missing, calculation shows that it must have been 13 Men 3 Zip. If the lower portion of the stela is ever found, one can predict that it will show the Sacred Round date 13 Men. In rare cases the Vague Year date was omitted, but if we ever find the year date on the missing half of Stela 29, it will be 3 Zip. In respect to its 365-day period, the vague year corresponds to our year most closely, and like ours it is divided into named months with numbered days of the months. But like the 360-day tun it lacked leap-days and so also falls behind our year, though much more slowly. This is a curious thing, for no one supposes the Maya failed to observe the slip of this vague year in the seasons.
It should be obvious that where one has Maya Long Count control, each date is fixed as so many tuns and days from every other date; if one such Maya date can be clearly correlated with a corresponding Christian date, all are potentially correlated with the proper Christian dates. When our Stela 29 date is said to fall in A.D. 292, that is according to a correlation theory which may be disputed. Choosing some other correlation theory would not affect the evidence that the Stela 29 Initial Series date was carved at Tikal before any of the known others, at Tikal or elsewhere.
